TB 9-4931-539-35
1 min = 60 s = 6 x 107 s
1 h = 3600 s = 3.6 x 109 s
1 d = 8.64 x 104 s
= 8.64 x 1010 s = 86400 s
1 s/min = 1.667 x 10 -8
1 s/h = 2.78 x 10 -10
1 s/d = 1.16 x 10 -11
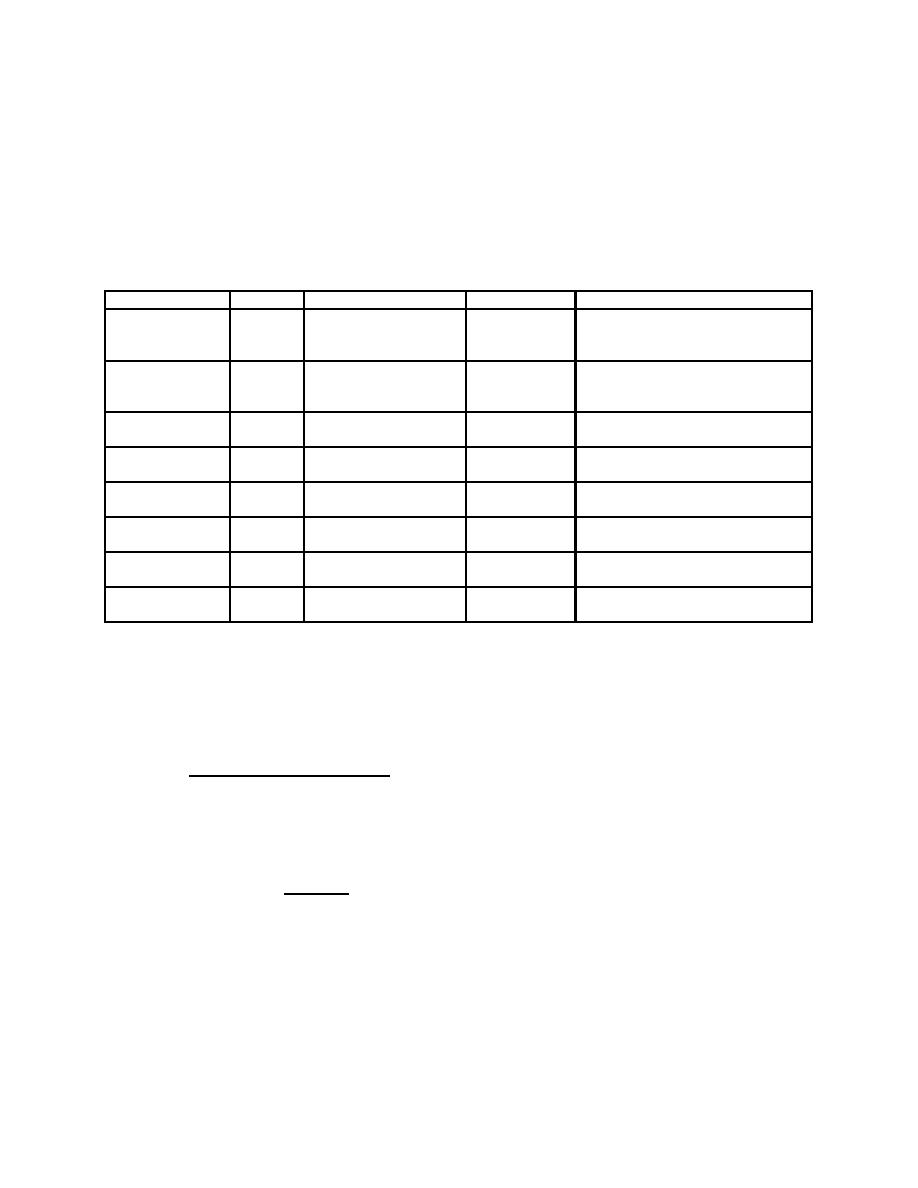
Table 2. DOD Omega VLF Radio Stations
Station
Letter
Operator
NORWAY
A
66 25 12.68 N
Valley span
NORWEGIAN
13 08 13.07 E
TELECOMMUNICATION
ADMINISTRATION (NTA)
LIBERIA
B
6
18 19.26 N
Grounded
LIBERIAN MINISTRY
10 39 51.85 W
1400' tower
COMMERCE, INDUSTRY,
AND TRANSPORTATION
KANEOHE HI
C
21 24 16.92 N
Valley span
U.S. COAST GUARD (USCG)
157 49 50.96 W
LA MOURE ND
D
46 21 57.40 N
Insulated
U.S. COAST GUARD (USCG)
98 20 08.22 W
1400'tower
LA REUNION
E
20 58 26.90 S
Grounded
FRENCH NAVY
ISL
55 17 23.62 E
1400'tower
ARGENTINA
F
43 03 12.79 S
Insulated
ARGENTINE NAVY
1500' tower
AUSTRALIA ISL
G
38 28 52.42 S
Grounded
AUSTRALIAN DOT
146 56 07.06 W
1400'tower
JAPAN
H
34 36 53.06 N
Insulated
JAPANESE MARITIME SAFETY
129 27 13.12 E
1500'tower
AGENCY (JMSA)
1WGS-84:
2 to 4 nautical mile accuracy, 95% confidence.
95% operating time for each station, including scheduled off air.
Three station availability worldwide 95% of the time.
No greater than +2 deviation of the phase transmitted signal from the synchronized mean.
The formula for calculating fractional frequency error is as follows:
FFE = difference in microseconds x 10-6
elapsed time in seconds
For example: If the MICROSECONDS counter reading is 5278.4 at 9:00 a.m. and 5240.1 at 1:30 p.m. of
the same day, the elapsed time is 4 h and 30 min, or 16,200 s. The net phase difference is 5278.4 minus
5240.1, or +38.3 s. The fractional frequency difference, then, is:
FFE
+38.3 x 10-6
= +2.36 x 10-9
16,200
The decrease in the MICROSECONDS counter reading in the example above indicates
that the local frequency standard is low in nominal frequency. Knowing this, you would
increase the frequency of the local oscillator. Consequently, an increase in the
MICROSECONDS counter reading would be indicative of an increase in nominal
frequency of the local standard. A decrease in frequency would be required on the local
5

